Zielorientierte adaptive Finite Elemente zur Parameteridentifikation konventioneller und additiver mikromorpher Kontinuumsmodelle

Bild vergrößern

Zusammenfassung

Aufgrund von Defiziten lokaler Kontinua, insbesondere der pathologischen Netzabhängigkeit von FE-Lösungen auf der numerischen Seite und der Simulation von längenskalenabhängigen Problemen auf der theoretischen Seite, haben generalisierte Kontinuumstheorien breite Anwendungsbereiche. In diesem Projekt befassen wir uns mit der Klasse der mikromorphen Kontinua, die mikropolare (Cosserat) Kontinua und Mikrodehnung-Kontinua als wichtige Sonderfälle enthält. Im vorigen Projekt haben wir die zielorientierte adaptive FEM für mikromorphe Elastizität und Plastizität behandelt, um das direkte Problem fehlergesteuert zu simulieren. Zusätzlich wurde ein neuer Kontinuumstyp vorgeschlagen, der als additives mikromorphes Kontinuum für die Elastizität großer Deformationen bezeichnet wird. Dabei werden ein flexibler Übergang zwischen verschiedenen Kontinua und deren Kombination mit verschiedenen Wichtungen ermöglich. Auf dieser Basis befasst sich dieses Projekt hauptsächlich mit folgenden Themen:

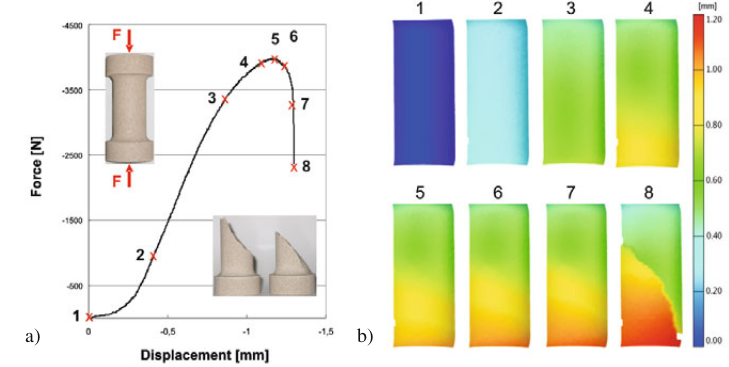
  • Bei konventionellen mikromorphen Theorien im Sinne von Eringen berücksichtigen wir Größeneffekte in der linearen Elastizität. Es werden neue Experimente zur Identifizierung von Parametern verwandter mikromorpher Modelle entwickelt, bei denen Kerben unterschiedlicher Größe verwendet werden, um Größeneffekte in Sandproben für das Cold-Box-Gießen zu aktivieren.
  • Das neuartige additive mikromorphe Modell wird um die Elastoplastizität in Kombination mit Schädigung erweitert. Da es als Sonderfälle mikropolare Kontinua und Mikrodehnung-Kontinua enthält, wird erwartet, dass die Auswahl eines geeigneten Kontinuums automatisch über ein inverses Problem erfolgt, das auf experimentellen Daten basiert. Dies wird anhand der Simulation des gesamten Schädigungsprozesses eines Cold-Box-Sandes veranschaulicht. Der Vorteil des neuen additiven Modells besteht darin, die Rotation von Sandpartikeln und die Verformung des Bindemittels mit geeigneten Wichtungen zu berücksichtigen.
  • Inverse Probleme zur Parameteridentifikation werden sowohl für konventionelle als auch für additive mikromorphe Modelle behandelt, die auf experimentellen Daten beruhen. Da ein heterogener Verformungszustand erforderlich ist, wird eine Sensitivitätsanalyse auf Basis diskretisierter Variationsformulierungen für die FEM durchgeführt. Besonderes Augenmerk wird auf das additive Modell mit zeitabhängigem Charakter gelegt.
  • Um die numerische Effizienz der Parameteridentifikation zu verbessern, wird eine adaptive FEM für eine effektive Vernetzung (räumliche und zeitliche Diskretisierung für zeitabhängige Probleme) entwickelt. Die Herausforderung besteht darin, geeignete zielorientierte Fehlerschätzer zu entwickeln, um die entsprechenden adaptiven Algorithmen zu steuern.
     

abstract

Due to deficiencies of local continua, in particular pathological mesh dependency of FE solutions on the numerical side and failure to simulate length scale dependent problems on the theoretical side, generalized continuum theories have wide areas of applications. In this project, we address the class of micromorphic continua, containing micropolar (Cosserat) continua and microstrain continua as important special cases. In the previous project, we dealt with goal-oriented adaptive FEM for micromorphic elasticity and plasticity towards an error-controlled simulation of the direct problem. In addition, a novel continuum type labeled as additive micromorphic continuum has been proposed for finite strain elasticity, enabling a flexible transition of different continua and weighting

them differently if required. On this basis, this project primarily addresses the following issues:

  • With conventional micromorphic theories in the sense of Eringen, we consider size effects in linear elasticity. New experiments will be designed for identifying parameters of related micromorphic models, where notches of varying sizes are used to activate size effects in sand specimens for cold-box casting.
     
  • The novel additive micromorphic model will be extended to elastoplasticity including damage. Since it contains micropolar and microstrain continua as special cases, a selection of a proper continuum is expected to be done automatically via an inverse problem based on experimental data. It will be illustrated by simulating the whole damaging process of a cold-box sand. The advantage of the new additive model is to account for the rotation of sand particles and the deformation of the binder with proper weights.
     
  • Inverse problems for parameter identification will be handled for both conventional and additive micromorphic models based on experimental data. As a heterogeneous deformation state is required, a sensitivity analysis will be carried out on the basis of discretized variational formulations for the FEM. Special care will be paid to the additive model with a time-dependent character.
     
  • To enhance the numerical efficiency of the parameter identification, adaptive FEM will be developed for an effective meshing (spatial and temporal discretization for time-dependent problems). The challenging part is to develop appropriate goal-oriented error estimators to drive the corresponding adaptive algorithms.
     

Veröffentlichungen

 

Förderinstitution

DFG, GZ: MA 1979/17-2